The shortest day of the year is the day of the winter solstice, which in 2024 is December 20 or 21 depending on time zone. It seems reasonable that the latest sunrise and earliest sunset would both occur on the shortest day. But it's not so; the earliest sunset is a couple of weeks earlier and the latest sunrise is a couple of weeks later. [Note 1] (The notes contain additional explanation that can be skipped on first reading.)
What explains this peculiarity? Why don't the earliest sunset and latest sunrise both fall on the shortest day?
The time of day when the sun reaches its highest point in the sky is called solar noon. The length of time from one solar noon to the next is called the solar day. That is, one solar day is the time it takes for the sun to return to its highest point in the sky.
The length of the solar day varies during the year: Near the winter and summer solstices the solar day is more than 24 hours long, and near the spring and fall equinoxes it's less than 24 hours long. (Why so? We'll see shortly.) Therefore: near the solstices, solar noon occurs at a slightly later clock time each day because the solar day is more than 24 hours and the clock day is always exactly 24 hours.
Next: Since solar noon is a little later each day, sunset is also a little later each day. (This is true because the time from solar noon to sunset doesn't change very much near the winter solstice [Note 2].) But if sunset is a little later each day, that means the earliest sunset has already happened! By the same reasoning, later solar noons at the solstice imply later sunrises, hence sunrise is getting later and the latest sunrise is yet to occur. [Notes 3, 4]
So now we see why a longer-than-average solar day causes both earliest sunset and latest sunrise to not fall on the solstice. The next step is to understand why the length of the solar day varies, and in particular why it is longer at the solstices.
There are two factors that change the length of the solar day. The first is that the earth's axis is tilted with respect to its orbit around the sun, and the second is that the earth's orbit is not a circle but an ellipse. The first of these factors, called the obliquity of the axis, has the larger effect and is the only one we examine here [Note 5]. So let's study the length of the solar day assuming that the tilted earth orbits the sun in a perfect circle.
Here's a summary: The length of the solar day is determined mostly by the rotation of the earth on its axis, but is also affected a little bit by the revolution of the earth around the sun. The rotation by itself would create a solar day of constant length whether the axis was tilted or not. The slight change caused by the revolution would be a constant if the earth were not tilted, but because of the tilt it is not constant; this effect varies through the year and makes the solar day longer near the solstices and shorter near the equinoxes. Now for the details. [The explanation that follows is based on a presentation by Andy Latto.]
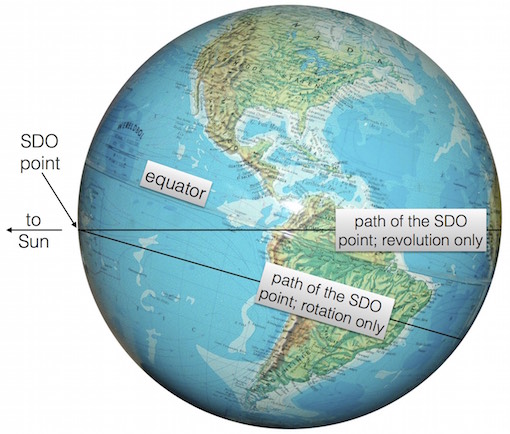
At any instant, there is exactly one point on the earth's surface where the sun is directly overhead. To find it, draw a line from the center of the sun to the center of the earth and stand on the point where the line touches the earth's surface. At that point, and no other, the sun is exactly overhead. It is solar noon at that point and also everywhere else at the same longitude.
If the earth were motionless with respect to the sun, the sun-directly-overhead point would always be in the same spot. But the point moves, both because of the rotation of the earth on its axis and because of the revolution of the earth around the sun. When the point moves once around the earth, one solar day has passed. When we say "once around the earth" we care only about the east-west motion of the point—solar noon recurs when the point traverses 360 degrees of longitude. The north-south motion of the point doesn't matter to us since it doesn't count in determining whether solar noon has arrived.
First let's examine the effect of the earth's rotation. Imagine an untilted earth not revolving around the sun, just rotating, and it's obvious that the sun-directly-overhead point moves along the equator, once around per day. Now mentally tilt the earth's axis and you'll see that the point follows some other parallel of latitude, still once around per day. [Note 6] Since the point moves along a parallel of latitude, it moves in a purely east-west direction, in fact due west. If we measure speed in degrees of longitude per hour (not in miles per hour!) we realize that the speed of the point is constant, no matter what latitude it's at; it goes once around, 360 degrees, each day.
The bottom line is that the rotation of the earth causes the sun-directly-overhead point to move directly west, crossing lines of longitude at a constant rate. If this were the only factor to consider, the length of the solar day would be constant regardless of any tilt.
Now we have to add the effect of the earth's revolution around the sun. Again, start by imagining the earth with untilted axis, this time not rotating but just revolving around the sun. You'll see the sun-directly-overhead point moving once around the earth each year. And (take my word for this) the point moves eastward, so its motion is contrary to the motion from the rotation—when added in, this motion makes each solar day a little longer than it would be from the rotation alone. (But just a very little, since the motion from the revolution is so much slower.) If the earth were not tilted, the motion due to revolution would be due east at constant rate, and would lengthen each day equally throughout the year.
But the earth is tilted! So the earth's revolution around
the sun doesn't move the sun-directly-overhead point along the equator, but
rather around a great circle that's tilted with respect to the
equator. Here's the path that the sun-directly-overhead point would
follow if only revolution around the sun was a factor (and also the
constant-latitude path that the sun-directly-overhead point would follow if
we only take into account the earth's rotation): 
Now the crux of the matter: As the sun-directly-overhead point moves around this great-circle path, it is not moving directly east. It moves generally east, but sometimes it's travelling a little northeast and sometimes a little southeast. It moves due east only at its most northerly and southerly extent, which happens at the solstices.
So even though the overall speed of the point is constant, the eastward component of the speed is not constant, and that's what we care about: it's fastest when the point is moving due east (at the solstices), and is smaller when the point is moving north or south as well as east. The eastward speed is slowest when the point has the largest northerly or southerly motion, that is, at the equinoxes, when the point crosses the equator. [Note 7]
Hence this once-around-per-year motion of the point, which makes each day a little longer, has a different effect at different times of the year. At the solstices the motion is due east, so its eastward motion is fastest and lengthens the solar day more. That is, at the solstices, the solar day is longer than its average length. At the equinoxes, the point is moving northeast or southeast as much as it ever does, so the eastward component of its speed is at its slowest and has the least effect at making the day longer. That is, at the equinoxes, the solar day is a little shorter than average. And we're done.
One last comment: If you take a picture of the sun each day when your clock says noon (not at local solar noon) the sun is not always in the same place in the sky. It moves east and west because of the variation in the length of the solar day, and it moves north and south with the change of the seasons. The combination of these motions traces out a figure-eight shape called the analemma which you can see pictured on some globes.
[1] The actual dates of earliest sunset and latest sunrise depend on latitude—near the equator, the earliest sunset occurs in November! Solstices and equinoxes happen at the same instant worldwide. back
[2] You might object to this: "The time between solar noon and sunset isn't constant!" I hear you cry. "It gets shorter in December and longer in July, making more or less daylight!" Yes it does, but that change happens very slowly near the solstices and can be ignored.
Think of it this way: There are two factors that change the time of sunset. The first is the change in the number of hours of daylight—we get fewer hours of daylight as we move towards December, which is reflected in earlier sunsets. The second factor is the difference between solar time and clock time, which we're explaining in this website (see Note 4). The first factor is the dominating factor through most of the year. Near the summer and winter solstices, though, the amount of daylight in the day is essentially constant, changing only a tiny amount from day to day, and the second factor comes into play. back
[3] We can apply the same reasoning to the summer solstice to conclude that the latest sunset must follow the summer solstice, and the earliest sunrise must precede the summer solstice. Just remember that at either solstice, both sunrise and sunset are getting later and you can always tell where the earliest/latest sunrise/sunset occur. back
[4] Here's another way to look at it: We don't tell time using the solar day, because we want all days to have exactly the same length. So we use clocks that run at a constant rate independent of the sun, ticking off exactly 24 hours each day. These clocks average out the variations in the solar day, making all days the same length, and so don't agree with the solar day. But when we ask about "earliest" sunset, of course we mean earliest according to the constantly-running clocks! The difference between clock time and true solar time creates the phenomenon we're investigating—if we used only sundials to tell time, the earliest sunset and latest sunrise would coincide with the winter solstice. back
[5] The second factor that changes the length of the solar day is called the eccentricity of the earth's orbit. Not only does it have smaller effect than the obliquity, it works the other way in summer—by itself, it would make the solar day shorter than 24 hours at the summer solstice. At the winter solstice, the obliquity and eccentricity work together to lengthen the solar day; at the summer solstice, they oppose each other. Since the effect of the obliquity is greater, the solar day is still longer at the summer solstice. But because the total effect is less, there are a couple of weeks between the earliest sunset and the winter solstice, but only several days between the latest sunset and the summer solstice. Go here to see the individual contribution of each factor to the length of the solar day. (The equation of time, the thing being graphed, is just the difference between solar noon and clock noon. Note that it's the slope of the equation of time, not its value, that's important to this discussion.)
By the way, it's much easier to understand why the eccentricity changes the length of the solar day. You can find an explanation at any number of websites where the eccentricity is incorrectly trotted forward as the sole reason that the earliest sunset isn't the same day as the solstice! back
[6] It's too bad that I don't have more visuals on this site; a picture is worth a thousand words and all that. If you'd like to help by creating graphics or animations to improve this page's explanations, winning undying honor and fame, please contact me. back
[7] The point doesn't move around the tilted circle at a truly constant speed, because the earth's orbit is not a perfect circle. The point moves slightly faster near perihelion (January) and slightly slower near aphelion (July). But the effect of this non-constant speed is less than the effect of the fact that the easterly speed varies depending on whether the point is going due east or northeast. In particular, the easterly component of the motion is at a near-maximum in July, because the fact that it is moving due east is more important than the fact that it's going a bit slower than usual. back
Copyright © 1997--2024 Larry Denenberg
Last modified 11/17/2024
Larry's home page